GRE Quantitative Reasoning - GRE Practice Online

Select Exercise Lists

By Question Type
Quantitative comparison
Numeric Entry
Word Problem
Data Interpretation

By Content
Arithmetic
Algebra
Geometry
Data Analysis

Overview and Practice of GRE Quantitative Reasoning

1 Overview

GRE general test includes a math-related section: quantitative reasoning. It evaluates the ability to reason quantitatively and to address problems with quantitative methods, or say necessary mathematical skills.

The questions are in two sorts:

Pure mathematical problems: answer by math knowledge only
Word problems: answer by modeling problems mathematically

The mathematical symbols, terminology, and conventions in the Quantitative Reasoning worksheets are understandable at the high school level. Besides, there are some other assumptions listed in the Quantitative Reasoning section directions:

All numbers used are real numbers.
All figures are in a plane unless otherwise indicated.
Geometric figures are not necessarily drawn to scale.
Coordinate systems are drawn to scale

You can use a calculator in the test, but you cannot use your own calculator. In the computer-based GRE test, the calculator is provided on-screen; as for paper-delivered test, the test center will offer a basic calculator. You may get latest and official information of GRE Quantitative Reasoning from Quantitative Reasoning Measure.

2 GRE Quantitative Reasoning

GRE Quantitative Reasoning isn't real math test. It doesn't test all high school math skills; for example, it does not include trigonometry, calculus, or other higher-level mathematics. Instead, it just covers four primary math parts: arithmetic, algebra, geometry, and data analysis.

Arithmetic Topics include properties and types of integers, arithmetic operations, exponents and roots, and concepts of estimation, percent, absolute value, the number line, and decimal representation.

Example:

Directions: Select a single answer choice or multiple choice.

10. An office has two copying machines Machine X can reduce the size of a page and produces copies, in either the reduced or original size, at the rate of 1 page per second. Machine Y cannot reduce pages and produces copies at the rate of 1 page every 2 seconds. What is the least amount of time required for the two machines together to make 60 copies of a 120 page document if exactly half of the pages of each copy of the report must be reduced?

	1 hour
	1 hour 20 minutes
	1 and 1/4 hour
	2 hours
	3 hours

Exercises of arithmetic topics

Algebra Topics include operations with exponents; algebraic expressions; equations and inequalities; linear and quadratic equations and inequalities; and coordinate geometry, including graphs of functions, intercepts and slopes of lines.

Example:

Directions: Enter your answer as an integer or a decimal if there is a single answer box OR as a fraction if there are two separate boxes — one for the numerator and one for the denominator.

A football team has won 10 games and lost 5 games. If the team wins the remaining games of the season, it will have won 80 percent of its games. How many games in total will have been played?

Exercises of algebra topics

Geometry Topics include parallel and perpendicular lines, circles, triangles, quadrilaterals, other polygons, three-dimensional figures, area, perimeter, volume, and angle measurement in degrees. (Not need to construct proofs of geometry.)

Example:

Directions: Select answer choice or choices.

Which of the following could be a value of x, in the diagram above?
Indicate ALL such values.

GRE quantitative test

	10
	20
	30
	40
	50

Exercises of geometry topics

Data Analysis Topics include basic descriptive statistics; interpretation of data in tables and graphs; elementary probability; conditional probability; random variables and probability distributions, including normal distributions; and counting methods, such as combinations and permutations.

Example:

Directions: Select a single answer choice or multiple choice.

Of the following, which is the greatest amount of money that a person can borrow at an annual interest rate of 6.5 per cent if the loan and interest are to be paid off at the end of one year with the money from a check of Rs. 1,600?

	Rs. 2,460
	Rs. 1,704
	Rs. 1,520
	Rs. 1,500
	Rs. 1,040

Exercises of data analysis topics

3 GRE Quantitative Reasoning Question Type

GRE Quantitative Reasoning has four common types of questions. A question may be promoted in two ways: either independently as a discrete question or as part of a set of questions, the latter case is usually in Data Interpretation whose all questions are based on the same data source, like tables or graphs.

Quantitative Comparison is the primary type in GRE quantitative reasoning and usually ask you select one answer choice from multiple options.

Exercises of quantitative comparison

Example:

Directions: Compare Quantity A and Quantity B, using additional information centered above the two quantities if such information is given, and select one of the following four answer choices:

Quantity A is greater.
Quantity B is greater.
The two quantities are equal.
The relationship cannot be determined from the information given.

A symbol that appears more than once in a question has the same meaning throughout the question.


A jar of 90 marbles contains marbles of three different solid colors: red, blue and green. More than half the marbles are either red or blue.

Quantity A	Quantity B

The number of blue marbles in the jar	Twice the number of green marbles in the jar

	Quantity A is greater.
	Quantity B is greater.
	The two quantities are equal.
	The relationship cannot be determined without further information.

Numeric Entry needs you calculate the answer; some Words Problem question also needs you give the numeric answer after modeling.

Exercises of numeric entry

Example:

Directions: Enter your answer as an integer or a decimal if there is a single answer box OR as a fraction if there are two separate boxes — one for the numerator and one for the denominator.

Sam travels from A to B at 10km/hr and returns at 15km/hr. Pam travels from A to B and returns at 15 km/hr.Pam takes 4 hours less than Sam does. What is the distance between A and B in kilometers?

Word Problem emphasizes to translate the problem to the mathematical model, you need to calculate the answer, select one or more choices from multiple options.

Exercises of word problem

Example:

Directions: Select answer choice or choices.

Which of the following can be used to illustrate that not all prime numbers are odd?

Select one or more of the choices.

	1
	2
	3
	4

Data Interpretation usually has a set of questions based on the same data source; the problems are diversity, like calculating the answer, selecting one or more choices from multiple options.

Exercises of data interpretation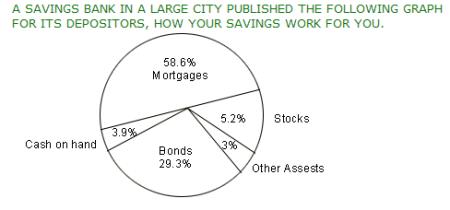

Example:

Directions: Questions 1 to 4 are based on the following data.

1. About how many degrees (to the nearest degree) are in the angle of the sector representing mortgages?

	59
	106
	211
	246
	318

2. The annual rate of interest from "other assets" is 4.8%. If the total assets of the bank are 57.6 million dollars, what is the annual income (in dollars) from "other assets"?

	82,944
	921,600
	1,728,000
	2,764,800
	3,600,000

3. The average annual interest on mortgage investments is m% and the average annual interest on the mortgage bond investment is b%. If the annual interest on the bond investment is x dollars, how many dollars are invested in mortgages?

	xm/b.
	xb/m.
	100xb/m
	bx/100m.
	200x/b

4. About how many degrees (to the nearest degree) are in the angle of the sector representing cash on hand?

	114
	14
	140
	39
	321